 |
International Union of Crystallography
|
Contributed by Massimo Nespolo
Special thanks to Prof. Slavomil Ďurovič
1. The OD theory
The theory of OD structures was developed in the fifties by Dornberger-Schiff to explain the disorder phenomena in λ-wollastonite and Madrell's salt. These structures, without being layered structures in the crystallochemical sense, can be considered as consisting of slabs with their own symmetry, containing coincidence operations constituting a diperiodic group (layer group) only within individual slabs. The symbol of a three-dimensional diperiodic group is the same as the symbol of the corresponding triperiodic group, but the direction of lacking periodicity is given in parentheses (Dornberger-Schiff, 1959). However, for the entire structure these coincidence operations are only local (partial), i.e. they are valid only in a subspace of the crystal space. The ambiguity (= existence of more than one equivalent possibilities) in the stacking of slabs arises from the existence of this local symmetry, which does not appear in the space-group type of the structure. The resulting structure can be "ordered" (periodic) or "disordered" (non-periodic), depending on the sequence of local symmetry operations relating pairs of slabs.
Dornberger-Schiff and co-workers developed a geometrical theory of these structures, which was called "OD theory", for "Order-Disorder". The building slabs themselves were called "OD layers". Clearly, OD phenomena are of completely different nature from the order-disorder phenomena in alloys. The OD theory is actually the symmetry theory of polytypism, but for quite a long time it passed almost unnoticed by polytypists, most likely because of the unfortunate name.
The set of all the operations valid in the whole crystal space constitutes a space group; by adding the set of all the operations valid in a subspace of it, one obtains a space groupoid (Dornberger-Schiff, 1964; Fichtner, 1977, 1979, 1980, 1986, 1988). The theory of groupoids was introduced in mathematics by Brandt (1927) and applied in crystallography in Germany by the OD school (Dornberger-Schiff, 1964, 1966), and in Japan by the school of Ito and Sadanaga (e.g. Ito, 1950; Ito and Sadanaga, 1976; Sadanaga, 1978; Sadanaga et al, 1980). The algebra of groupoids is a quite complex subject and it is not treated here. Readers interested in this subject can consult the references at the end of this page.
2. Definition of OD structures
In the OD theory, a central role is played by the vicinity condition (VC), which states the geometrical equivalence of layer pairs. The vicinity condition (see, e.g., Dornberger-Schiff, 1979) consists of three parts:
- VC α: VC layers are either geometrically equivalent or, if not, they are relatively few in kind
- VC β: translation groups of all VC layers are either identical or they have a common subgroup
- VC γ: equivalent sides of equivalent layers are faced by equivalent sides of adjacent layers so that the resulting pairs are equivalent.
If the position of a layer is uniquely defined by the position of the adjacent layers and by the VC, the resulting structure is fully ordered. If, on the other hand, more than one position is possible that obeys the VC, the resulting structure is an OD structure and the layers are OD layers. VC structures may thus be either fully ordered structures or OD structures (Dornberger-Schiff, 1964, 1966, 1979; see also Ďurovič, 1999). All OD structures are polytypic; the reverse may or may not be true (see the arguments in Zvyagin, 1993). Equivalency depends on the choice of OD layers and also on the definition of polytypism.
All OD structures, even of different substances, built according to the same symmetry principle, belong to an OD groupoid family: this is an abstract family, whose members are the groupoids describing the symmetry of the substances sharing the same symmetry principle. Then, the OD structures of the same substance built on the same structural principle belong to one and the same family: the members of a family are individual, real structures (Ďurovič and Weiss, 1986).
3. Classification of symmetry operations
For analyzing the polytypism of layer compounds, two types of symmetries must be recognized:
- λ-symmetry: the symmetry proper of an individual layer
- σ-symmetry: the symmetry of a layer pair
The symmetry operations acting on layers are then divided into four types:
- λ-operations: symmetry operations transforming a layer into itself; the set of λ-operations constitute a diperiodic group (layer-group)
- σ-operations: coincidence operations transforming a layer into the adjacent one
- τ-operations: symmetry or coincidence operations which do not change the sign of the coordinate in the layer stacking direction. They are labelled λ-τ or σ-τ if they refer to λ- or σ-operations, respectively
- ρ-operations: symmetry or coincidence operations which change the sign of the coordinate in the layer stacking direction, and thus turn a layer or a stack of layers upside down. They are labelled λ-ρ or σ-ρ if they refer to λ- or σ-operations, respectively. Evidently, τ.τ = τ, τ.ρ = ρ, ρ.τ = ρ and ρ.ρ = τ.
4. The example of the close packing of equal spheres: the NFZ relation
(after Ďurovič, 1997)
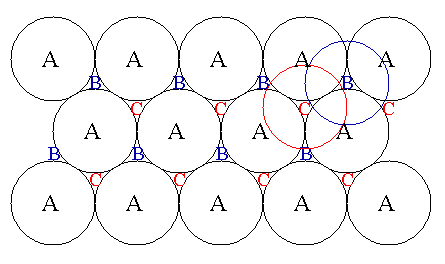
Fig. 1. Geometry and notation of close packing of equal
spheres. If the first layer is labelled A, two types of triangular
voids, B and C, with opposite orientation, exist. Positions indicated by the
same letter are translationally equivalent, whereas positions indicated
by different letters are not.
The diperiodic group of a single layer of spheres is P(6/m)mm, order 24. However, only a subset of these 24 operations remain valid for a pair of layers, and this subset can be obtained by analyzing, for simplicity, the τ-operations.
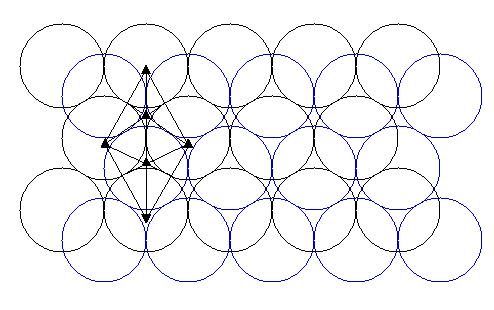
Fig. 2. Analysis of the τ-symmetry
of a pair of layers of equal spheres. τ-operations
with a continuation: 1, 62(=31), 64(=32),
3m. τ-operations without a
continuation: 61, 63(=2), 65, 3m
If N is the order of the τ-diperiodic group, and F that of its subgroup which contains the operations valid for a pair of layers (operations which "have a continuation in the adjacent layer"), the number Z of positions of the adjacent layer leading to geometrically equivalent layer pairs is given by the NFZ relation:
Z = N/F = 12/6 = 2
There are thus two ways, geometrically equivalent, of stacking the second layer. In fact, spheres of any layer can be placed in all the voids B or in all the voids C, which produce two possible geometrically equivalent pairs of adjacent layers, AB and AC (any reflection planes perpendicular to the layers and passing for the centres of A transform B into C and vice versa). Although Z is immediately evident in a simple case like that of of close packing of equal spheres, the NFZ relation applies equally to more complex cases, where Z may be far less evident. Besides, the above analysis holds not only for close packing of spheres, but also for any conceivable structure built by layers and layer pairs with the same symmetry.
4. The OD layers and the OD packets
The OD interpretation presupposes that any polytype of a given polytypic substance may be considered as consisting of disjunct parts periodic in two dimensions, called OD layers, whose pairs remain geometrically equivalent in any polytype of the same family. The OD layers do not necessarily coincide with the layers commonly chosen on the basis of the chemical identity and/or cleavage properties. In other words, the layers by which a polytypic substance is most commonly described from the crystal-chemical point of view are not always the most suitable layers to describe the geometrical equivalence of layer pairs. Furthermore, the choice of the OD layers in general is not absolute (Grell, 1984); their purpose is not to explain but to describe and/or predict polytypism of a substance based on symmetry.
The crystal chemical reason for polytypism is that adjacent layers (two-dimensionally-periodic units) can be linked to each other in many translationally non-equivalent ways. However, the nearest-neighbor relationships remain preserved. Translated into the language of symmetry, this means that the pairs of adjacent layers remain geometrically equivalent in all polytypes of the same family.
The geometrical equivalence must be fulfilled not necessarily by the real layers, but by their archetypes, i.e. the (partially) idealized layers to which the real layers can be reduced by neglecting some distortions occurring in the true structure. The notion of polytypism becomes thus unequivocal only when it is used in an abstract sense to indicate a structural type with specific geometrical properties.
In dealing with OD structures built by M > 1 kinds of layers, it is useful to introduce a structural unit bigger than an individual OD layer: the OD packet. An OD packet is the smallest continuous part of an OD structrue, which is periodical in two dimensions and which represents completely its composition. Any OD structure can be thought of as consisting of a sequence of OD packets (Ďurovič, 1974)
5. MDO polytypes
Any family of polytypes contains theoretically an infinite number of periodic and non periodic structures. The periodic polytypes are divided into two groups, on the basis of number of kinds of layer triples.
- MDO (Maximum Degree of Order) polytypes: contain the smallest possible number of layer triples (when equivalence of all layers triples is possible, this number is 1).
- Non-MDO polytypes: contain more kinds of layer triples than the minimal number geometrically possible.
MDO polytypes include the most frequently occurring polytypes which have been termed "basic", "standard", "regular" etc. by the experimentalists. All these definitions are phenomenological and not really systematic, whereas MDO is a geometric concept.
The reason for the MDO polytypes being the most frequently occurring ones is easy to understand. Since the interatomic forces decrease rapidly with the distance, as a first approximation only the forces confined within a distance corresponding to a pair of layers need to be taken into account. But all pairs of adjacent layers are geometrically equivalent in all polytypes of a given family (definition of OD structure). As a result, all polytypes of a given family should be energetically equivalent. In practice, however, longer-range interactions, although weaker, are not completely negligible, especially for compounds built by thinner layers. Triples like, e.g., ABA and ABC are, in general, energetically non-equivalents, because they are geometrically non-equivalent. Polytypes containing the smallest number of layer triples are evidently also energetically favored. These simple considerations explain why, among the infinitely many possible ordered polytypes, MDO polytypes are by far the most frequent.
Clearly, the above reasoning holds rigorously only under two conditions:
- geometrical abstraction: layers are replaced by layer archetypes
- layer-by-layer growth
The true layers are more or less distorted and the geometrical equivalence is no longer rigorously respected. In some cases these distortions may make certain non-MDO polytypes energetically favored over others MDO polytypes (this is the case of non-MDO subfamily A polytypes vs. MDO subfamily B polytypes in micas). Besides, the energy difference between an MDO polytype and a non-MDO polytype are small, and in any case other factors, especially of kinetic nature, dominate the polytype formation in the phase when the crystal is growing by spiral growth. Notwithstanding, the frequency of occurrence of MDO polytypes shows unequivocally that, despite they represent an approximation, energetic considerations based on the MDO concept is capable of explaining the prevalence of certain polytypes in many cases.
6. Superposition structures, family structure and family reflections
By superposing two or more identical copies of the same polytype translated by a superposition vector (i.e. a vector corresponding to a submultiple of a translation period) a fictitious structure is obtained, which is termed a superposition structure. Among the infinitely possible superposition structures, that structure having all the possible positions of each OD layers is termed a family structure: it exists only if the shifts between adjacent layers are rational, i.e. if they correspond to a submultiple of lattice translations.
The family structure is common to all polytypes of the same family (Dornberger-Schiff, 1964; Ďurovič, 1994). From a group-theoretical viewpoint, building the family structure corresponds to transforming ("completing" all the local symmetry operations of a space groupoid into the global symmetry operations of a space-group (Fichtner, 1977, 1980). Additional "virtual atoms" are created by the completed operations, and the resulting model may have physically unrealistic interatomic distances: they appear in the superposition structure, which is a purely mathematical construction, as a consequence of the group-theoretical process of completing the local symmetry operations.
The group of translations of the polytype reciprocal lattice can be decomposed into a subgroup of translations, which corresponds to the Fourier transform of the family structure (family sublattice), and one or more cosets. The family sublattice is again common to all polytypes of the same family. This means that all polytypes of the same family, normalized to the same volume of scattering matter, have a weighted sublattice in common. The diffractions that correspond to the family sublattice are termed family diffractions (or, more commonly, family reflections). When indexed with respect to the basis vectors of any of the polytypes of the same family, the family sublattice shows several non-space-group absences, which indicate the existence of local symmetry operations. Clearly, the family reflections convey important information, because they reveal the symmetry of the family structure.
The family reflections are always sharp, including the case of non-periodic (disordered) polytypes. In fact, the disorder of the stacking concerns the distribution of subsequent ρ-operations. If this distribution is periodic, after a finite even number of steps a period is closed and the product of those ρ-operations is the generating τ-operation (the product of an even number of ρ-operations is a τ-operation). If instead the distribution of subsequent ρ-operations is not periodic, no generating τ-operation can be found, and the polytype is disordered.
In the family structure the ρ-operations are completed to global operations: the family structure and its Fourier transform, which consists in the family reciprocal sublattice, are thus common to both periodic and non-periodic polytypes of the same family (Ďurovič and Weiss, 1986; Ďurovič, 1997, 1999). The remaining diffractions, which correspond to the cosets of the weighted reciprocal lattice with respect to the family sublattice, are termed non-family reflections and are instead typical of each polytype: they can be sharp or diffuse, depending on whether the polytype is ordered or not, i.e. on whether the distribution of subsequent ρ-operations is ordered or random.
Because the family structure can be deduced from the symmetry principle of the polytype family, it is possible to illustrate its derivation by means of a very simple, hypothetical example, in which the actual atomic arrangement is not taken into account, and geometrical figures with the appropriate λ-symmetry are used instead.
Let us consider the three hypothetical polytypes (Ďurovič, 1999) and their geometric diffraction patterns in Figure 3.
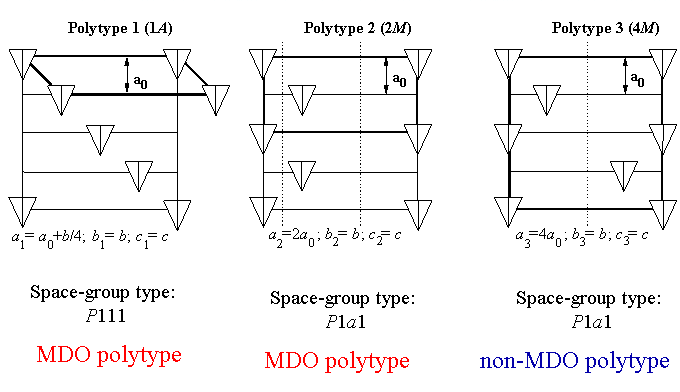
Fig. 3. Three hypothetical polytypes built by stacking a
layer with
λ-symmetry [.m.]
(represented by isosceles triangles)
These polytypes are constructed by stacking equivalent layers perpendicular to the plane of the drawing, with λ-symmetry P(1)m1. The stacking direction is a, and the distance between adjacent layers is |a0|. The λ-symmetry is indicated by isosceles triangles with a mirror plane [.m.]. The three polytypes can be related to a common orthogonal four-layer cell with a = 4a0, inside which the cell of the polytype is shown by bold lines (Fig. 3).
The first polytype (1A, MDO) has basis vectors a1 = a0 + b/4; b1= b; c1 = c and space-group type P111. The only global τ-operation is the translation a0 + b/4.
The second polytype (2M, MDO) has basis vectors a2 = 2a0; b2= b; c2 = c and space-group type P1a1. The global τ-operations are the translation a = 2a0 and an a-glide plane at y = 1/8 and 5/8.
The third polytype (4M, non-MDO) has basis vectors a3 = 4a0; b3= b; c3 = c and space-group type P1a1. The global τ-operations are the translation a = 4a0 and an a-glide plane at y = 0 and y = 1/2.
The geometric diffraction pattern of each of these polytypes can be divided into two parts (Fig. 4): k = 2k (open circles) and k = 2k+1 (full circles).
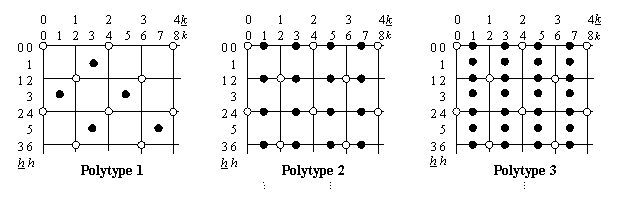
Fig. 4. Geometrical diffraction pattern corresponding to
the three polytypes in Fig. 3. h and k are
the indices in the reciprocal sublattice common to the three polytypes.
The k = 2k are the family reflections, which define the family reciprocal sublattice, common to all the three polytypes. The Fourier transform of this subgroup of diffraction gives the family structure (Fig. 5), with space-group C1m1, a = 2a0, b = b/2: the superposition vector is b/2.
The non-family reflections are those for which k = 2k+1: the number of reflections along each row in the four-layer reciprocal cell is the same as the number of layers in the period of the polytype. (Nespolo and Ďurovič, 2002)
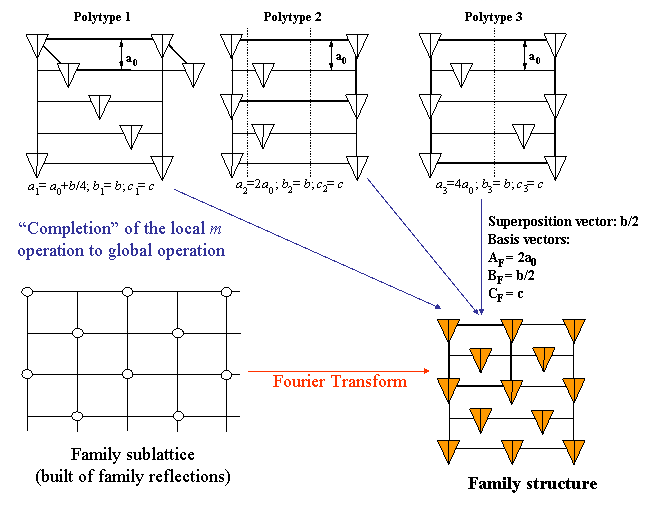
Fig. 5. Derivation of the family structure by completion
of local symmetry operations to global symmetry operations (blue),
superposition of polytypes, and Fourier Transform of the family
sublattice (red).
For polytypes with period longer than one layer, the rows containing family reflections (family rows), when indexed in the cell of the polytype, show systematic absences which cannot be explained by the space-group symmetry. Those absences derive, in fact, from the local symmetry operations.
When a twin operation by merohedry leads to superposition, along the family rows, of reflections "present" from one individual with reflections "absent" from another individual, an alteration of the geometry of the diffraction pattern is observed, despite the purely merohedric character of the twin. This type of twinning is called twinning by selective merohedry.
Bibliography about OD structures, space groupoids and polytypism (only works of general character are listed)
Brandt H (1927) Über eine Verallgemeinerung des Gruppenbegriffes. Mathematische Annalen 96, 360-366
Brown R. (1987) From Groups to Groupoids: a Brief Survey. Bulletin of the London Mathematical Society, 19: 113-134.
Dornberger-Schiff K (1956) On Order-Disorder Structures (OD-Structures). Acta Crystallographica 9: 593-601
Dornberger-Schiff K (1959) On the nomenclature of the 80 plane groups in three dimensions. Acta Crystallographica 12, : 173
Dornberger-Schiff K (1964) Grundzüge einer Theorie von OD-Strukturen aus Schichten. Abh dtsch Akad Wiss Berlin, Kl f Chem 3, 107 p
Dornberger-Schiff K (1966) Lehrgang üer OD-strukturen. Berlin: Akademie-Verlag 135p
Dornberger-Schiff K (1979) OD structures - a game and a bit more. Kristall und Technik 14: 1027-1045
Dornberger-Schiff K, Grell-Nieman H (1961) On the theory of Order-Disorder (OD) Structures. Acta Crystallographica 14: 167-177
Dornberger-Schiff K, Fichtner K (1972) On the Symmetry of OD-Structures Consisting of Equivalent Layers. Kristall und Technik 7: 1035-1056
Dornberger-Schiff K (1982) Geometrical Properties of MDO Polytypes and Procedures for their Derivation. I. General Concept and Applications to Polytype Families Consisting of OD Layers All of the Same Kind. Acta Crystallographica A38: 483-491
Dornberger-Schiff K, Grell H (1982) Geometrical Properties of MDO Polytypes and Procedures for their Derivation. II. OD Families Containing OD Layers of M > 1 Kinds and Their MDO Polytypes. Acta Crystallographica A38: 491-498
Dornberger-Schiff K, Grell G (1982) The concepts of "crystal", "OD crystals" and "MDO crystal". Soviet Physics: Crystallography 27: 73-77
Ďurovič S (1974) Notion of "packets" in the theory of OD structure of M>1 kinds of layer. Examples: Kaolinites and MoS2. Acta Crystallographica B30: 76-78
Ďurovič S (1979) Desymmetrization of OD structures. Kristall und Technik 14: 1047-1053
Ďurovič S (1994) Significance of superposition structures in the polytypism of phyllosilicates. In Aperiodic '94 Proc Int'l Conf on Aperiodic Crystals. G Chapuis, W Paciorek (ed) World Scientific, Singapore / New Jersey / London / Hong Kong, ISBN 981-02-2080-4, p 595-599
Ďurovič S (1997) Fundamentals of OD theory. In Modular aspects of minerals / EMU Notes in Mineralogy, vol. 1. S Merlino (ed) Eötvös University press, Budapest, p 1-28
Ďurovič S (1999) Layer stacking in general polytypic structures. Sect. 9.2.2 in International Tables for Crystallography, Vol. C (Ed. ACJ Wilson, E Prince) Dordrecht / Boston / London: Kluwer Academic Publishers, 752-765
Ďurovič S, Weiss Z (1986) OD structures and polytypes. Bulletin de Minéralogie 109: 15-29
Ferraris G, Makovicky E, Merlino S (2008) Crystallography of Modular Materials. IUCr monographs on crystallographyNo. 15, IUCr-Oxford University Press
Fichtner K (1977) Zur Symmetriebeschreibung von OD-Kristallstrukturen durch Brandtsche und Ehresmannsche Gruppoide. Beiträge zur Algebra und Geometrie 6: 71-99
Fichtner K (1977) A new deduction of a Complete List of OD-Groupoid Families for OD-Structures Consisting of Equivalent Layers. Kristall und Technik 12: 1263-1267
Fichtner K (1979) On the Description of Symmetry of OD Structures (I). OD Groupoid Family, Parameters, Stacking. Kristall und Technik 14: 1073-1078
Fichtner K (1979) On the Description of Symmetry of OD Structures (II). The Parameters. Kristall und Technik 14: 1453-1461
Fichtner K (1980) On groupoids in crystallography. MATCH, communications in mathematical and in computer chemistry 9: 21-40
Fichtner K (1986) Non-space-group symmetry in crystallography. Computers & Mathematics with Applications. 12: 751-762
Fichtner K (1988) Order-disorder structures. Computers & Mathematics with Applications. 16: 469-477
Fichtner-Schmittler H (1979) On Some Features of X-Ray Powder Patterns of OD Structures. Kristall und Technik 14: 1079-1088
Grell H, Dornberger-Schiff K (1982) Symbols for OD Groupoid Families Referring to OD Structures (Polytypes) Consisting of More Than One Kind of Layer. Acta Crystallographica A38: 49-54
Grell H (1984) How to choose OD layers. Acta Crystallographica A40: 95-99
Ito T (1950) X-ray Studies on Polymorphism. Maruzen Co., Tokyo, 231 pp
Ito T, Sadanaga R (1976) On the crystallographic space groupoids. Proceeding of the Japan Academy 52: 119-121
Nespolo, M. (2008) Does mathematical crystallography still have a role in the XXI century? Acta Crystallographica A64: 96-111.
Nespolo, M. and Ďurovič, S. (2002). Crystallographic basis of polytypism and twinning in micas. In Mottana, A., Sassi, F.P., Thompson, J.B.Jr., Guggenheim, S. (Ed.): Micas: Crystal Chemistry & Metamorphic Petrology. Reviews in Mineralogy and Geochemistry / Mineralogical Society of America, 46: 155-279
Sadanaga R (1978) Complex structures and space groupoids. Recent Progresses of Natural Sciences in Japan (ISSN 00800295) 3: 143-151
Sadanaga R, Sawada T, Ohsumi K, Kamiya K (1980) Classification of superstructures by symmetry. Journal of the Japanese Association of Mineralogists, Petrologist and Economic Geologists, Special Issue No. 2: 23-29
Weinstein A (1997) Groupoids: Unifying Internal and External Symmetry. Notices of the American Mathematical Society, 43: 744-752
Zvyagin BB (1993) A contribution to polytype systematics. Phase Transitions, 43: 21-25

